Upper elementary students must master place value in order to understand large numbers, and visual models can play a pivotal role. Grasping the base ten system is a big cognitive step from the one-to-one correspondence of counting and ordinance. By the fourth grade, students are working with numbers so large that counting is completely impractical. They must understand groups of ten, one hundred, and beyond by learning the value of each place in the base ten system.
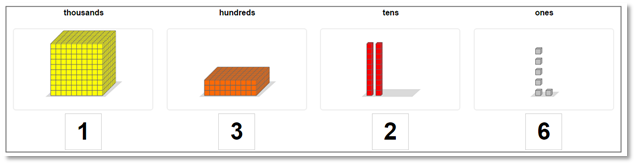
Dynamic visual models can help children build number sense around place value. Base Ten Blocks are a common hands-on manipulative, and their virtual counterparts are excellent tools. A quick look at the representation of 1,326 shows why these on-screen blocks are regarded as leaning towards the concrete end of the abstraction spectrum: they are proportional and manipulating them on screen is similar to working hands-on. The digital models can link with numeric and text representations.
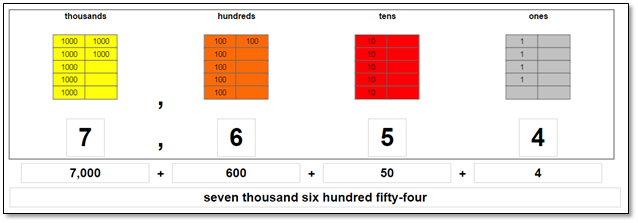
The Base Ten Frame provides a way to organize sets of 1, 10, 100, and 1000 into quickly-recognizable patterns. This model bears a relationship to the traditional ten frame and dot cards used to build number sense with small values. The Base Ten Frame, used here to represent the number 7,654, moves developmentally beyond Base Ten Blocks by using numerals as part of the representation. The combination of the ten frames, the place value labels, and the standard notation shown in the example build a context for relationship between expanded notation and standard notation. 7 thousands yields a value of 7,000. And, if you have 7,000 then you also have 7 thousands. Everything makes sense.
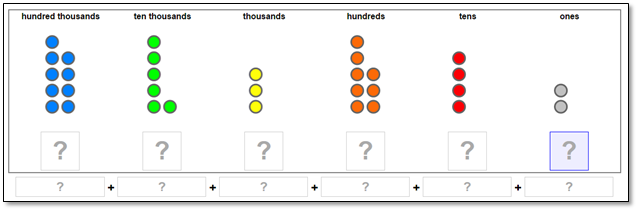
The third model uses Base Ten Disks, also known as Base Ten Coins or Singapore Disks, to represent number up to 1,000,000. This model calls upon the student to mentally combine the place value label with corresponding disks to discern the number. Colors are provided for consistency and comfort. The cognitive demand is higher than the other two models and comes with the benefit of representing larger numbers. (Note that Base Ten Blocks inherently top out at 1,999.) In the Conceptua Math product, from which these pictures are copied, the numbers can be “unlinked” or disengaged from the models. The Base Ten Disks shown here are not linked to the standard or expanded notation, leaving question marks in locations where students can enter the corresponding numbers.
When all three models are faded and the visual supports withdrawn, a student relies upon a word sentence, standard notation, and/or expanded notation to fully describe large numbers. The scaffolded range of visual representations builds cognitive connections, and the student gains the number sense required to master place value. That is the VALUE of place value MODELS.
