I want to share four important strategies for using number lines effectively in upper elementary grades. I have been concerned about the use of the number line, especially in upper elementary grades, because I’ve seen mixed results. There are times when I’ve seen teachers and students using the number line very effectively. And there are other times where it seems that the use of the number line can lead to complicated results and a lot of frustration.
In order to dig deeper, I embarked on a simple research project. I reviewed thousands of pages of content from Engage New York, and I searched for specific references to the number line in the upper elementary grades of three through five. I chose Engage New York because it’s available online, it’s free of charge, and I can use digital document reviewing techniques to quickly scan for references to the number line.
I discovered that there are hundreds and hundreds of representations and uses of the number line. After extensive study, I came away with some very clear conclusions. In order to share my observations more easily, I’ve created a rapid fire video with almost 300 screenshots of materials I studied. Before I move on to my observations and conclusions, I invite you to watch and see what I saw. Take a look, and see if you agree with my conclusions.
My observations are as follows:
Observation one: Number lines are used to address many content areas. I found uses of the number line in telling time, identifying fractions, operations with whole numbers and decimals, coordinate graphing, liquid and solid measurement, and just about every other topic that is taught throughout grades three through five.
Observation two: There are many different instructional designs that are all called by one name, the Number Line. Some number lines are horizontal, others are vertical, and there are even some on the diagonal. Some are calibrated with tic marks that clearly partition a range between a starting value and a terminal value. Others are not calibrated at all, and invite the student to freely indicate their sense of spatial relationship (open number lines). Still others are not calibrated and yet expect a student to pinpoint the discrete location that identifies the value of a number relative to starting points and ending points. You get the idea, and certainly I could go on. We all understand that there’s great flexibility in the use of the number line. Yet I think we need to be sensitive to the fact that this dramatic flexibility can also lead to complexity for the student. The number line is a highly varied model.
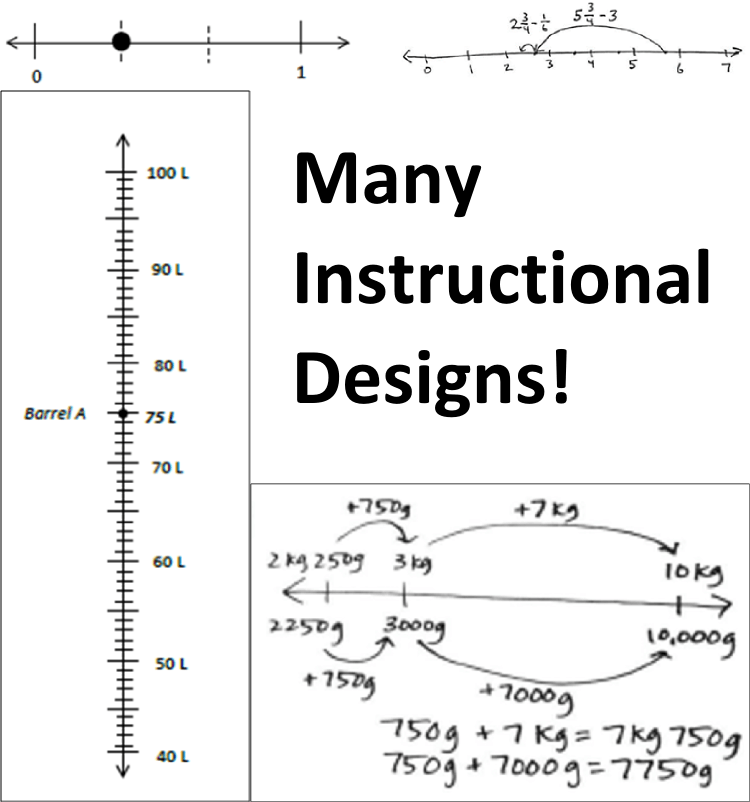
Observation three: Sometimes the number line is used as a representation for thinking, and other times as a representation of thinking. When the number line is used as a representation for thinking, it becomes a cognitive device that contributes to problem solving. This is certainly the case in many uses of the open number line in which a student is essentially using pencil and paper to create an artistic work that helps to visualize a solution. In this case, they are learning by using the number line. At other times the number line is used as representation of thinking, or is essentially a quiz. When the student is shown a list of values in order to plop them on a number line there may be no active learning value for the student, while there may be an assessment value for the teacher or curriculum that gave the order. In this case, they are showing what they already know by using the number line.
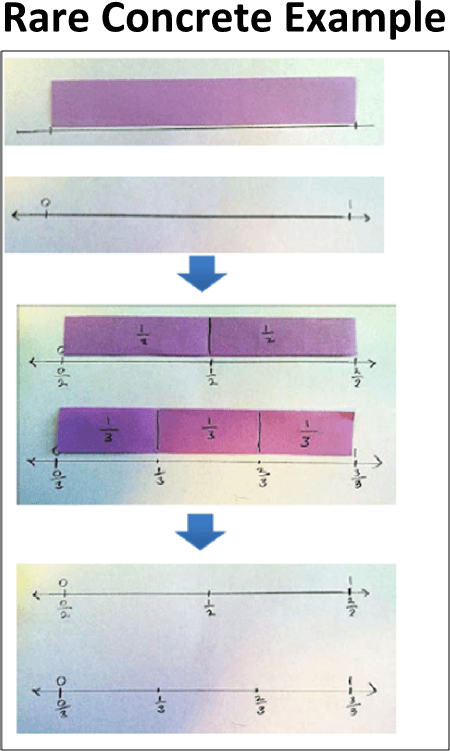
Observation four: On the concrete to representational to abstract spectrum, the number line often leans in the direction of abstraction. In the rare instances where a number line closely models some kind of concrete representation, as one might see in a number line that is paired with fraction strips, the number line is somewhat concrete. If a number line is clearly shown to divide a whole value into equal parts, it too may represent a cognitive model for the part/whole relationship of a fraction. But, much more often than not, the number line is quite abstract. So, while we as adults might consider the number line to be a visual model, the student may be seeing the model as a complicated abstraction for them to have to report definitive findings.
These observations lead me to the following recommendations for educators:
Recommendation #1: Be very clear about the topic that you are addressing when using a number line. If you choose to present number lines that represent different ranges of numbers (0 to 10, then 5 to 15, then 103 to 113), be very certain that your instructional objective is fully about the comprehension of those number ranges. If it’s simply to ensure that a student understands the value of numbers, you might be placing a layer of cognitive trickery on the child that isn’t helpful. As another example, if you are teaching the relative value of fractions that have common numerators, and you want to clearly show how the location of 2/3 is dramatically different from 2/30 on a number line, then your marriage between content and representation may be very appropriate.
Recommendation #2: Use a number line whose instructional design matches your content. If you are teaching part-whole relationships in fractions, you want to use a number line that segments the whole into equal parts. If you are teaching the concept of repeated addition as a method for solving multiplication problems, a series of vectors placed above the horizontal number line can be extremely helpful. But having students place discrete dots on a number line to precisely locate the value of a fraction or the result of a repeated addition may have no value at all.
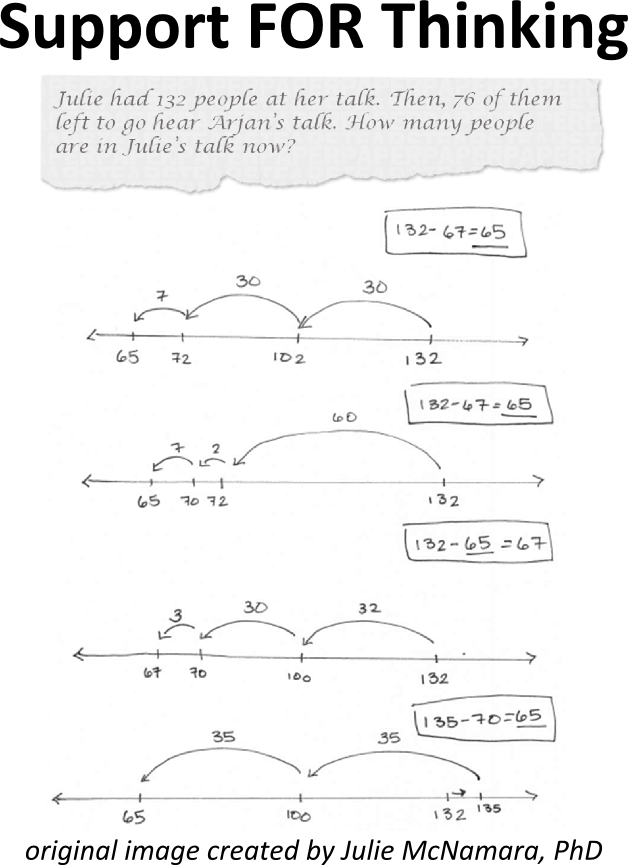
Recommendation #3: Whenever possible, use number lines as a support FOR thinking rather than a representation OF thinking. Many of us have discovered the joy of open number lines and bar models (closely related to number lines) over the past few years. Why? Because we realize that these are cognitive aids for both the student and ourselves. Additionally, they help reveal the students’ thinking. For instance, consider two open number lines that are used to solve subtraction problems. These problems employ the same set of numbers, yet from a Cognitively Guided Instruction perspective they represent different thinking processes. The beauty of the open number line is that I can reveal those varying solutions.
Recommendation #4: If the number line is too abstract for your students, be prepared to go to the left. To the extent that the C – R – A sequence can be seen as going from left to right, be prepared to back up one step to the left. If the student doesn’t understand something when you’re using a number line, you may need to introduce a more concrete manipulative. In the instance of the subtraction problems previously referred to, you may need to bring out linking cubes in order to engage that child. If the part-whole relationship of fractions doesn’t ring true on the number line, the child may be more successful with vertical fraction bars or even a fraction pie.
These four suggestions should help you unravel the number line for your students. I have personally spent hundreds and hundreds of hours over the last five years designing number line interactions for the Conceptua Math tools and curriculum. I invite you to take a look at the many different approaches to number lines that are presented through Conceptua Math.